Alexandre Grothendieck, a committed genius

11.29.2024, by

Alexandre Grothendieck (1928-2014) during an algebraic geometry seminar in Bure-sur-Yvette during the 1960s.

Alexandre Grothendieck, who is considered one of the founders of modern algebraic geometry, left a considerable mark on mathematics through his genius and his reflections on his time. Ten years after his death, the mathematician Leila Schneps revisits his legacy.

You and the mathematicians and former activists that knew Alexandre Grothendieck (1928-2014) decided to create the Grothendieck Circle in order to collect his archives. How did this collective wish emerge?
Leila Schneps.1 In1991, Esquisse d’un programme2 (Sketches of a programme) fell into my hands, and then Récoltes et Semailles3 (Harvest and sowing). I really wanted to read other texts by this author. I learned that a number of people had letters in their possession, or notes, or had simply met and shared thoughts with Grothendieck. One day in 2003, we met in a café in Paris and decided to create the Grothendieck Circle to gather all of the documents in one place. There was the mystery of this man who appealed to all of us, and we especially knew that there were tons of mathematics that had not yet been published. That is how we began to sift through and analyse this work that had remained anonymous for so long. I think that every member of the group ultimately wanted to gain a deep understanding of his highly distinctive legacy.

Grothendieck is especially known for his work on algebraic geometry. Yet his career as a mathematician began with a dissertation on analysis, a discipline that focuses on mathematical functions. Is he recognized for this work?
L. S. Yes, undeniably. There were three major mathematical periods in his career, and the first indeed involved functional analysis. Specialists in this field affirm that his work had a massive impact, and that the objects he introduced continue to be used today. His thesis directors, Laurent Schwartz4 and Jean Dieudonné, even said that “he killed the subject,” given how well he identified and responded to the numerous questions he was asked.

Alexandre Grothendieck (at left) discussing with Alain Schwartz. His work on algebra and geometry earned him the Fields Medal in 1966 (Bure-sur-Yvette, 1960s).

What mathematical objects did he introduce in his thesis?
L. S. In this first phase of his work, he focused on functional analysis. Let us remember that a function is a mathematical object that transforms elements from set X into elements from set Y. Functional analysis is notably the study of all the functions involved in the transformation of set X into set Y. They are spaces that are somewhat different than those we are accustomed to, as they do not consist of points or curves, but rather functions. Schwartz and Dieudonné wrote an article on a particular space, which they provided with a new structure known as topology . Schwartz wanted to extend this natural topology to general cases, and presented this problem to Grothendieck as a thesis subject. Grothendieck was disappointed at first, because he found two natural topologies instead of one, without one appearing better than the other. It was later that he understood that these two topologies coincided on the space that was of interest to Schwartz. So he introduced the notion of nuclear space to refer to spaces on which the two topologies cohabit.

In 1954, he “met” algebraic geometry and abandoned functional analysis. Why was this emerging discipline so appealing to him?
L. S. At the time, the discipline was not entirely new, but it was clearly in the process of changing. Originally, algebraic geometry is the study of curves, surfaces–or similar objects of larger size known as “varieties”–defined by one or more equations. However, in the early 1950s, the “new” algebraic geometry was born in France, in which the traditional notion of varieties was replaced by the notion of “schemes” (in French). Rather than use equations to describe a variety, it is associated with fairly complex mathematical objects that facilitate the precise measurement of its properties. If we know these objects sufficiently well, we can free ourselves from the initial object, and concentrate on this new representation. When Grothendieck discovered this theory, he saw it as an incredibly powerful tool. It allowed him to see mathematical questions from a new angle, and to more easily find solutions to the problems he was trying to solve. This was the beginning of the second phase of his work.

The mysterious archives of the mathematician Alexandre Grothendieck—70,000 handwritten pages produced between 19 August 1992 and 24 August 2014—were stored in the cellar of a bookstore in the 6th arrondissement of Paris.

His work in algebraic geometry earned him the Fields Medal in 1966. What innovations did it provide in the discipline?
L. S. He had already made major contributions to laying the foundations for this “new algebraic geometry.” The elements he introduced in his 1957 book Sur quelques points d'algèbre homologique (Some aspects of homologic algebra)5 are so well-known and widely used that it is difficult to say it “only” dates from 70 years ago. This is very recent for mathematics. Incidentally, this period was highly fruitful for Grothendieck: between 1955 and 1970 he produced an absolutely incredible amount of mathematics, including very difficult theorems that resisted mathematicians. However, he was especially known and recognised for the major structures he introduced, such as topoi.

Could you describe what topoi are?
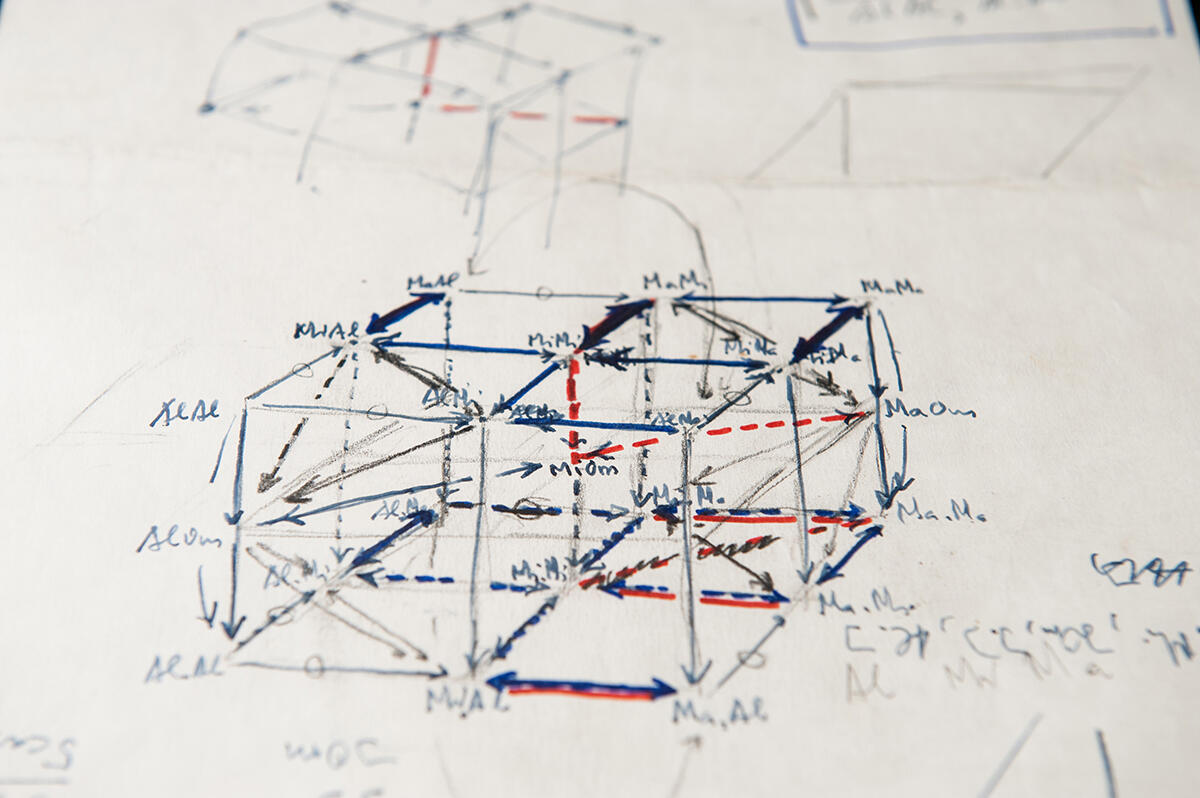
L. S. With topoi, Grothendieck applied the traditional notion of space while changing the definition of the point. Each point in space is now associated with a very complicated object that measures the properties of the space in relation to this point (for example, it will look at what is happening in the space immediately surrounding it). The point thus becomes a very rich object. The set of these points and the associated measurements ultimately make it possible to forget the original space. What is henceforth important are these points, and the amount of information they contain. They enable very precise measurements for the properties of varieties. A colleague, the Italian mathematician Olivia Caramello, proposed an interesting popularisation for these objects by describing them as the mathematical incarnation of Grothendieck’s idea of “vision,” which is to say having multiple viewpoints of a subject. Topoi can be summarised as a kaleidoscopic object that embodies all of the possible viewpoints for a same theme or variety.

© Université de Montpellier, IMAG, côte n°19

Handwritten notes on topoi, made between 1958 and 1979, and conserved at l’université de Montpellier.

Why did Grothendieck introduce these structures?
L. S. For him–and perhaps more so than for any other mathematician–it was important to create bridges between different subjects. I would go so far as to say that he saw the various areas of mathematics (arithmetic, geometry, analysis, etc.) as so many languages able to describe a given object. Going from one language to another allowed him to shed new light and to demonstrate theorems that would otherwise be out of reach. Topoi are an example of objects that, for Grothendieck, should build a bridge between arithmetic and geometry.

Was this bridge ultimately built?
L. S. While we have made progress, one could not say that this link has been established as Grothendieck envisioned. He was unable to pursue his idea further for lack of time, and also because he was expected–and he expected of himself–to develop his machinery until the end in order to prove theorems. Since the 1970s, numerous mathematicians from different domains have used his work on topoi, which sheds new light and leads to unexpected results. However, nobody has been able to approach what Grothendieck had in mind, firstly because he did not indicate, in a sufficiently clear manner, what he believed was the best way to unify geometry and arithmetic using topoi. Also, he had a vision that was naturally very abstract, yet it is not always simple for mathematicians to separate themselves from what is concrete for them.

Front pages from the journal Survivre… et vivre (Survive…and live) created by Grothendieck in 1970 in Montreal.

He was also a very politically committed mathematician. In 1966, he refused to go to the USSR to receive the Fields Medal out of solidarity for two writers who were dissidents of the Soviet regime. The following year, he spent multiple weeks in Vietnam to protest the intervention of the United States. One could say that he was not afraid to raise an antimilitarist voice. Was it rare at the time to be so committed?
L. S. He had highly committed friends such as Claude Chevalley and Pierre Samuel . However, that was not the case for the majority of the community. Few mathematicians were concerned about environmental issues, for instance, and even less by military ones, or at least very few were as activist as he. Grothendieck was not afraid of taking a firm stand, or of what people thought of him. For him, war, the atomic bomb, poverty, and the destruction of living beings were the most concerning.

In 1969, he decided to leave l’Institut des hautes études scientifiques where he worked after discovering that the institution was partly funded by the Ministry of Defence. Aside from this position, did the positions he adopted have repercussions on his career?
L. S. Yes, undeniably. In the beginning, he tried to involved the mathematical community in his movements. For example, he often told the story of how he housed an undocumented Japanese monk. This was a misdemeanour in the eyes of the French justice system, for which he received a suspended jail sentence and paid a fine. Shortly afterwards, he went to the very prestigious Bourbaki seminar that was being held in Paris, not to present his most recent discoveries or reflections, but to explain what he had just lived through, and to ask those who wanted to fight this law to join him. By the end of his intervention, almost the entire audience had left the room. This shows how much political commitment as a scientist was not commonplace at the time. He also tried, on multiple occasions, to convince researchers to reflect on the consequences of their work, notably its militarisation, and especially those stemming from mathematics. But the result was the same, a failure. It was at this point that Grothendieck felt especially rejected by his community–and he was effectively rejected. He was very sad, deeply affected, wounded almost.

© Archives Grothendieck/ Droits Réservés

Grothendieck in Rue Polonceau in the 18th arrondissement of Paris, August 1975. Posing in front of the temple of the Nichidatsu Fujii Guruji order, between Gaston Galaon (left) and the Japanese monk Oyama (right). Behind him are Morito and Chantal, Gaston’s daughter and wife.

After this break with his peers, Grothendieck left Paris and settled between Montpellier and l’Ariège. Did this move mark the end of his mathematical work?
L. S. At the time, he genuinely considered stopping mathematics, but was unable to do so. He said it himself: at certain moments, the ideas came flowing into his mind. The years between 1970 and 1991 marked his third major period of research, one that was also rich. He wrote major manuscripts containing fine mathematics, but his research during this period was much less known and appreciated, for it did not consist of publishable writings. They were more like scribbled ideas that succeeded one another without definition or theorem. He had freed himself from the time of “tasks,” which is to say the complete demonstrations and verifications that had filled his days before 1970. I find his late work magnificent: it is more mysterious, he goes where his vision takes him.

Were there still subjects that drew his curiosity at the time?
L. S. They were subjects that he would have liked to explore before, but he was too busy with his “tasks.” For instance, there is a 40-page typescript entitled Esquisse d’un programme (Sketches for a Programme) that I found in 1991, seven years after it was written. I had to work very hard to get into it, especially as very few people were working on it. In short, it was a research project that Grothendieck presented to members of the CNRS, for he was eyeing a post so he would not have to teach anymore. The primary topic of this project is the absolute Galois group, the central object of number theory. In his presentation, he explains how to study it in an entirely new way–not through number theory but through geometry.

These trunks, which were found after his death, held thousands of pages of the mathematician’s work.

You said that his work did not have much impact. Did this change over time?
L. S. This was true, but it is changing. Three or four of these manuscripts have been published (at least partially) since the 1980s. By “published” I mean proofread, understood, and annotated. Some of them were already available in the form of typed manuscripts, but others were available only in the Montpellier archives, as Grothendieck had left them in 1991 to his former student and friend Jean Malgoire, who later sent them to l’université de Montpellier. For example, the manuscript for Les dérivateurs (Derivators)6 took longer to be discovered, because it was hidden and nobody was able to lay eyes on it–and of course to study it–for decades. Many others remain.

As time went by, Grothendieck lived secluded in a village in l’Ariège, often refusing visits. You were able to meet him and exchange with him at the time. What did that change for you?
L. S. My encounter with Grothendieck–first through his writing, and later through our exchanges–was essential for me. He was, in a way, was my teacher of mathematical thinking by virtue of his ideas, his approach, and the questions he raised. In mathematics, we all fear not succeeding, failing, being wrong, investing a ton of time for nothing. Grothendieck made me aware of this fear in me. He taught me to fight it by allowing the simple joy of discovery to rise up within me. Thanks to this, I allow myself to seek out the joy of exploration much more often.

Footnotes